Author:
(1) Yitang Zhang.
Table of Links
- Abstract & Introduction
- Notation and outline of the proof
- The set Ψ1
- Zeros of L(s, ψ)L(s, χψ) in Ω
- Some analytic lemmas
- Approximate formula for L(s, ψ)
- Mean value formula I
- Evaluation of Ξ11
- Evaluation of Ξ12
- Proof of Proposition 2.4
- Proof of Proposition 2.6
- Evaluation of Ξ15
- Approximation to Ξ14
- Mean value formula II
- Evaluation of Φ1
- Evaluation of Φ2
- Evaluation of Φ3
- Proof of Proposition 2.5
Appendix A. Some Euler products
Appendix B. Some arithmetic sums
15. Evaluation of Φ1
Recall that Φ1 is given by (13.8). In view of (12.2), B(s, ψ) can be written as
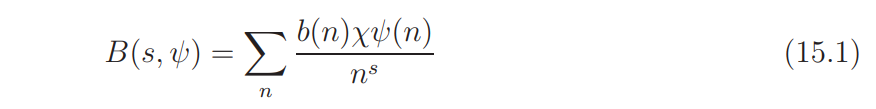
with
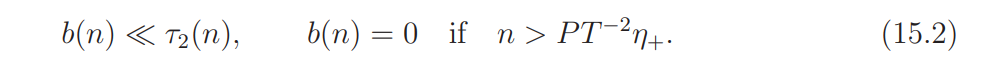
Write
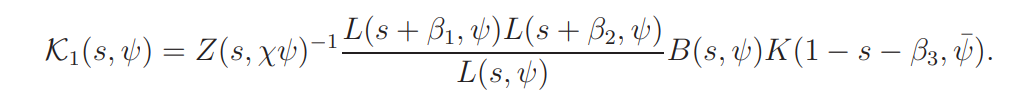
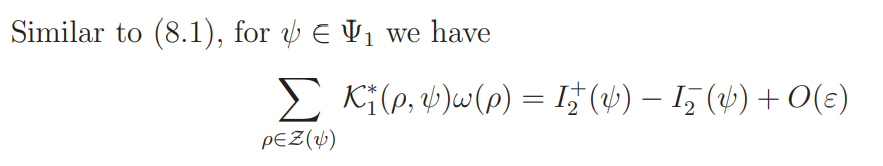
where
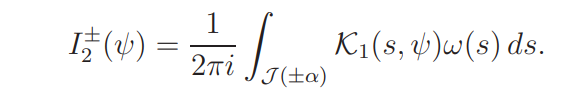
Hence
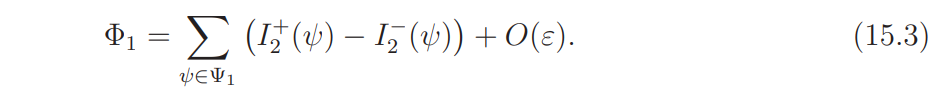
First we prove that

Since
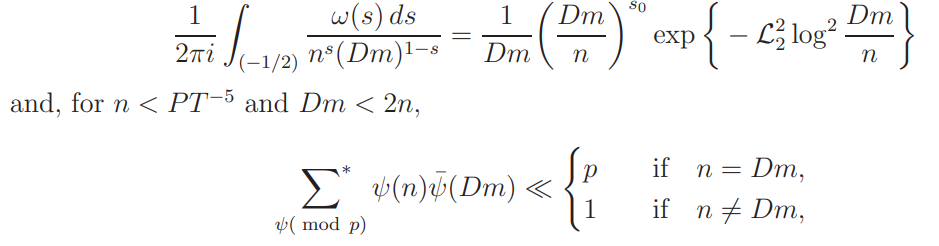
it follows that
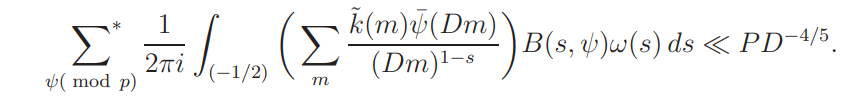
This yields (15.4).
Let κ1(m) be given by
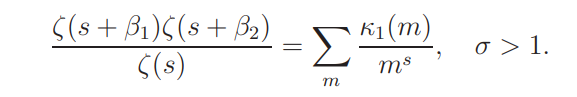
Regarding b as an arithmetic function, for σ > 1 we have
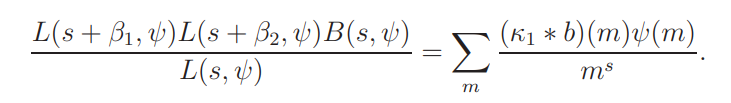
On the other hand, we can write
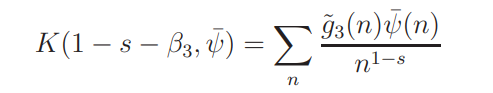
with
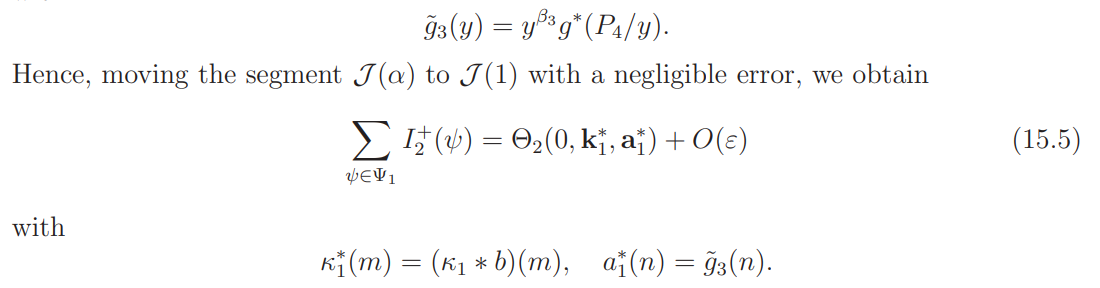
It follows by (15.3)-(15.5) and Proposition 14.1 that

where
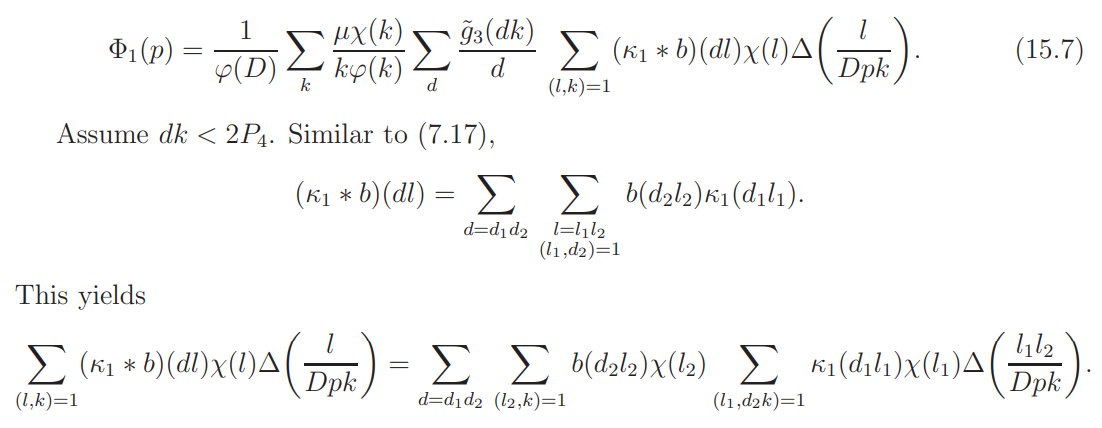
The innermost sum above is, by the Mellin transform, equal to
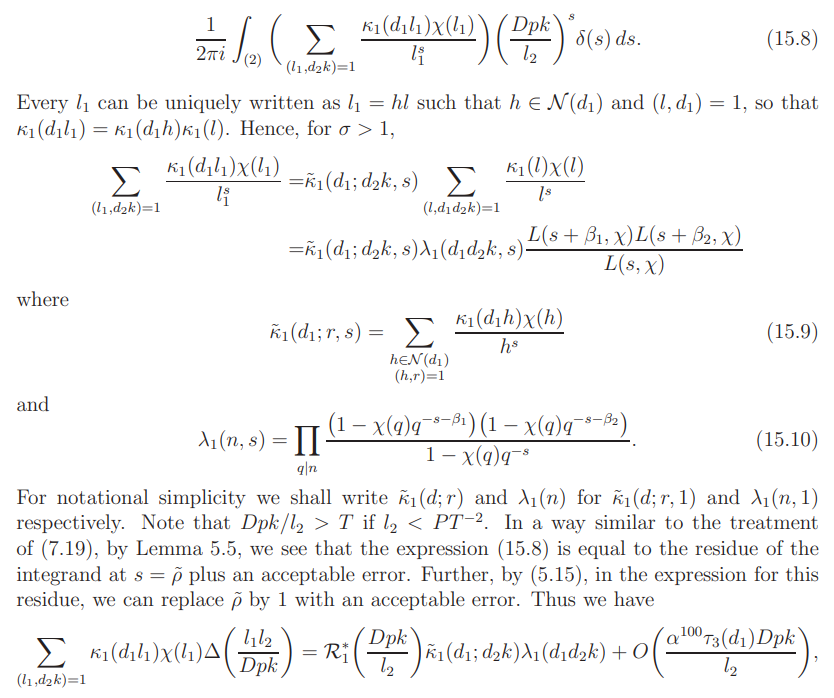
where
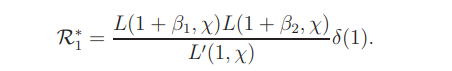
This yields
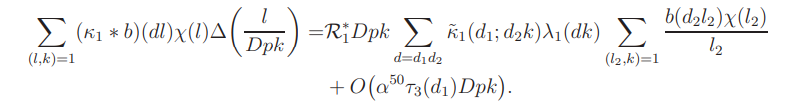
Hence
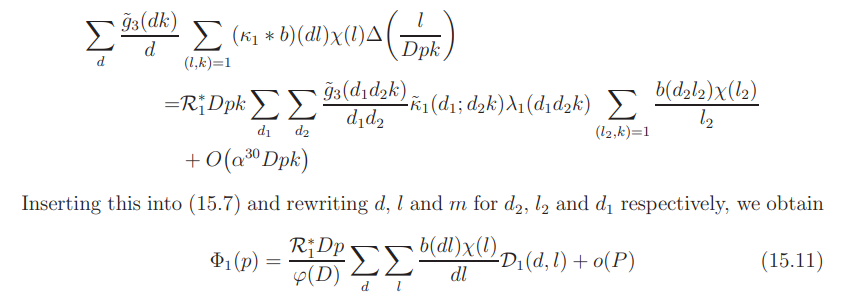
where
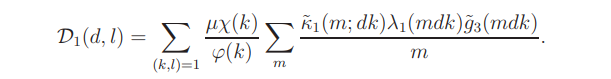
On substituting n = mk we can writ
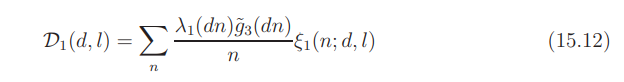
with
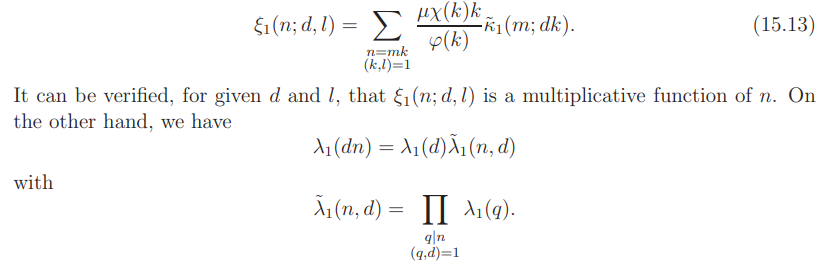
Hence
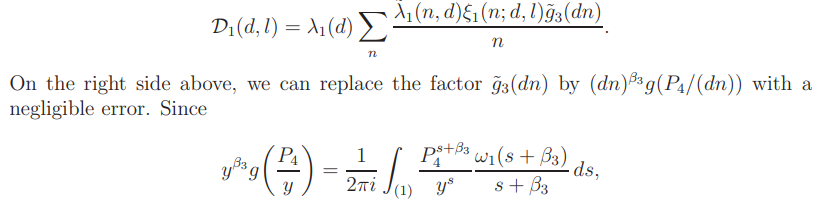
it follows that
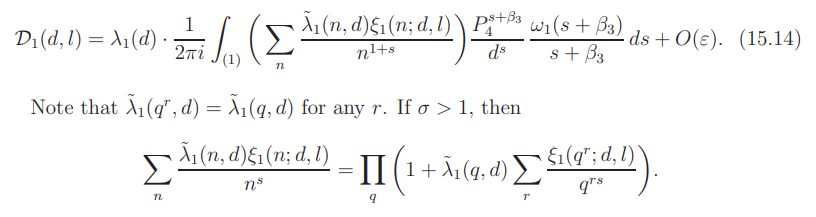
If (q, dl) = 1, then
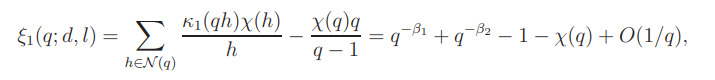
so that
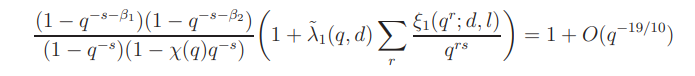
for σ > 9/10. In case (q, dl) > 1 and σ > 9/10, the left side above is trivially
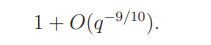
It follows that the function
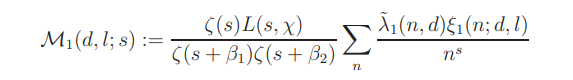
is analytic and it satisfies
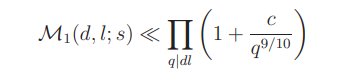
for σ > 9/10. The right side of (15.14) can be rewritten as

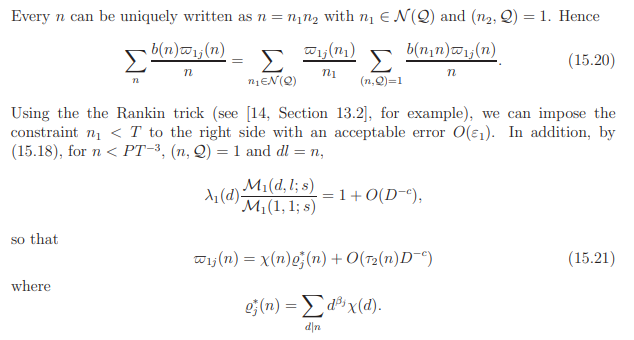
The following lemma will be proved in Appendix B.
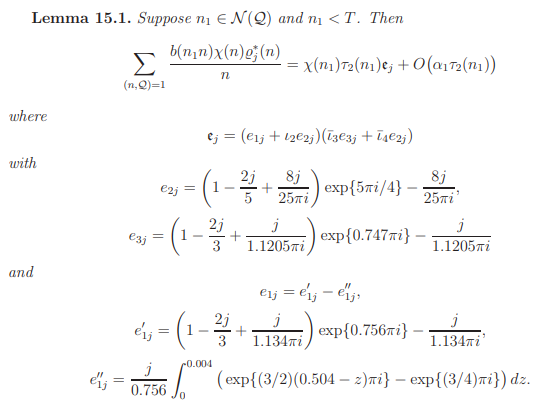
By (15.19)-(15.21) and Lemma 15.1 we obtain
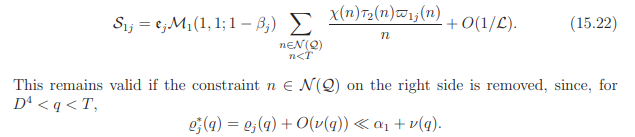
This yields, by (15.21),
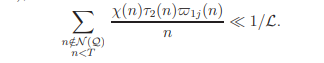
To apply (15.22) we need two lemmas which will be proved in Appendix A.
Lemma 15.2. If |s − 1| < 5α, then
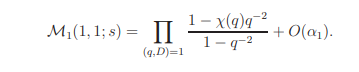
Lemma 15.3. For σ ≥ 9/10 the function
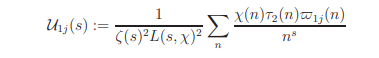
is analytic and bounded. Further we have
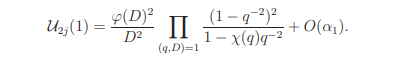
By (4.2) and (4.3),
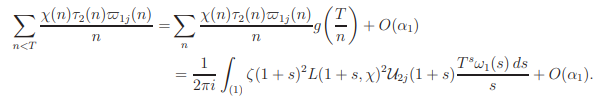
By Lemma 15.3, we can move the contour of integration in the same way as in the proof of Lemma 8.4 to obtain
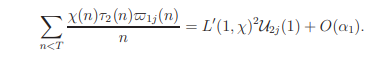
This together with Lemma 15.2 and 15.3 yields
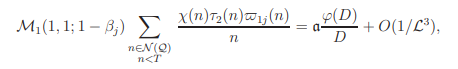
since
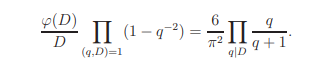
It follows by (15.22) that
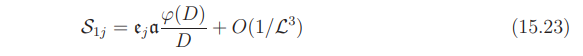
By Lemma 5.8,
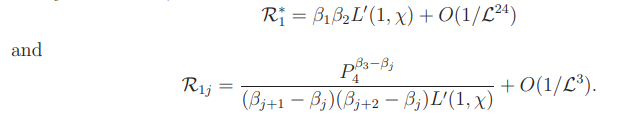
Hence, by direct calculation,
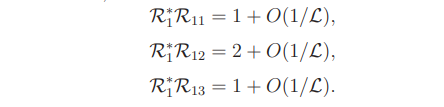
Combining these relations with (15.23) , (15.17) and (15.6) we conclude

This paper is available on arxiv under CC 4.0 license.

